virahAnka, hEmachandra, Fibonacci, sOnA patterns

The title I gave may look a bit strange. It will become obvious after some time. virahAnka was a Sanskrit prosodist who lived during the sixth or seventh century. Prosody is the science and art of writing poetry. Prosody basically can be divided in two ways - akshara and mAtra. akshara Chandassu depends upon a series of long and short syllables in a line. mAtrA Chandassu depends on syllabic instants. The time taken to utter a short syllable like ma is one mAtra (I) or kala and the time to utter a long syllable like maa is two mAtrAs (U or S). virahAnkA's expertise and erudition was revealed in the mAtrA Chandassu.
1 mAtra - I, 2 mAtrAs - II, U, 3 mAtrAs - III, UI, IU, 4 mAtrAs - IIII, UII, IUI, IIU, UU, mAtrAs - IIIII, UUI, UIU, IUU, IIUI, UIII, IIIU, IUII, and so on. This becomes obvious in singing too. For example, where mAdhavA occurs, we can also say muraharA without upsetting the rhythm or sarasijA for sarOjA, etc. If we examine the above numbers 1, 2, 3, 5, 8, etc. carefully, we have 1+2=3, 2+3=5, 3+5=8, 5+8=13, 8+13=21, etc. The same numbers occur for the jAtis in the tALa scheme also in music. Later in the twelfth century, hEmachandra sUri, a great Jain monk, elaborated on the same scheme in great detail. hEmachandra (from the present Gujarat area) was called sarvaj~na (the all knowing) in those days. An award also is given in his name today. In the beginning of the thirteenth century, Fibonacci of Pisa, Italy proposed the same numbers and they are today called Fibonacci numbers. Alas, the contributions by virahAnka and hEmachandra are forgotten and rarely mentioned! There is an intrinsic relationship between these numbers and the golden ratio. Wherever there is growth, this series and the irrational number phi occurs. It is beyond the scope of this blog to dwell upon it. But there is plenty of information on the net. Read, enjoy and get educated.

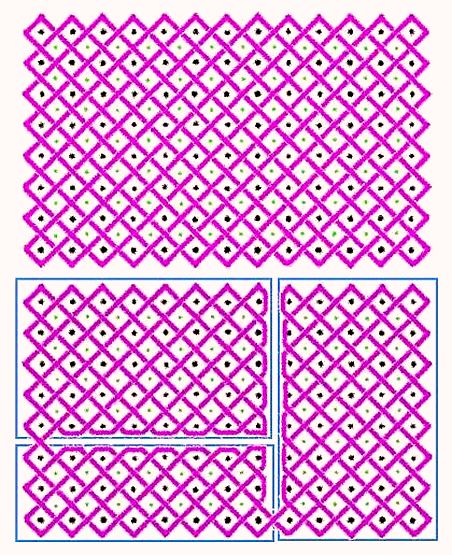
The purpose of this blog is to examine the sOnA patterns with the row and column numbers as two successive virahAnka numbers, say 5 and 8. These numbers are relatively prime and so we get a single line. I was wondering how a sOnA pattern like this can be further subdivided in to smaller sOnA patterns but with similar row and column numbers. I found that I could do it with three smaller sOnA patterns with virahAnka numbers inscribed in the bigger sOnA pattern. This is always the case. If the bigger sOnA pattern has c rows and d columns (in this example c=5, d=8), the smaller sOna patterns have the previous numbers as their row and column numbers. In this case, they are two sOnA patterns with 3 and 5 (or 5 and 3) and one with 2 and 5. Thus we have four successive virahanka numbers a, b, c, d for which a+b=c, b+c=d, then cd=2bc+ac. Actually the product cd is the total number of black dots and bc is the number of black dots in the smaller rectangles and ac is the number of black dots in the smallest rectangle.

In the figure for illustration, I chose c=8, d=13 and in the sOnA pattern, I have a single line. I have three sOnA patterns in blue rectangles (5x8, 8x5, 3x8, cd=104, 2bc=2x40=80, ac=24). I have opened up the top so that there is only line. With this pattern, if a sOna square is created, it will have two lines. I'll illustrate this property in the regular uploads.

What I have described is quite novel and this gives the connection between Fibonacci numbers and sOnA patterns. Enjoy!

Regards! - mOhana